Accurate initialization of islanded microgrid including induction motor load using unified power-flow approach

Abstract

An increased integration of distributed generators into microgrids presents a technical challenge to maintain voltage and frequency stability, especially in islanded or autonomous operation. For stability assessment of the microgrid, a proper initialization is particularly needed to get the right steady-state operating point when the widely used induction motor (IM) loads are mainly considered. Since a conventional power-flow approach is not straightforward and suitable for initializing the IM loads in the isolated microgrid network due to an unavoidable discrepancy between initial-bus scheduled and actual values of IM’s reactive powers. To eliminate this mismatch, this paper presents a precise power-flow initialization using a unified Newton–Raphson approach. The correct steady-state initializations are demonstrated using 6bus and 13bus microgrid systems. IEEE standard 33, 38, and 69bus distribution networks are employed to explore computational performances of the proposed algorithm for the case where a large number of IM loads are incorporated via distribution feeder loads.

Appendices

Appendices

1.1 Appendix A

This subsection provides some detail of Jacobian derivation. For the initializing method I, the Jacobian $\textbf{J}({\textbf{x}})$ in (22) can be obtained by making partial derivatives of $\textbf{f}({\textbf{x}})$ as,

$$\textbf{J}({\textbf{x}})={\textbf{J}_1}+{\textbf{J}_2}$$

(25)

where

$$ {\textbf{J}_1} { = }\left[ {\begin{array}{*{20}c} {\frac{{\partial {\mathbf{P}}_{{g}}^{{{cal}}} }}{{\partial {{\textbf{x}}}}}} & {\frac{{\partial {\mathbf{P}}_{{L}}^{{{cal}}} }}{{\partial {{\textbf{x}}}}}} & {\frac{{\partial {\mathbf{P}}_{{m}}^{{{cal}}} }}{{\partial {{\textbf{x}}}}}} & {\frac{{\partial {\mathbf{Q}}_{{g}}^{{{cal}}} }}{{\partial {{\textbf{x}}}}}} & {\frac{{\partial {\mathbf{Q}}_{{L}}^{{{cal}}} }}{{\partial {{\textbf{x}}}}}} & {\frac{{\partial {\mathbf{Q}}_{{m}}^{{{cal}}} }}{{\partial {{\textbf{x}}}}}} & 0 & 0 \\ \end{array} } \right]^{{{T}}} $$

$$ {\textbf{J}_2} { = } - \left[ {\begin{array}{*{20}c} {\frac{{\partial {\mathbf{P}}_{{g}}^{{{sch}}} }}{{\partial {{\textbf{x}}}}}} & {\frac{{\partial {\mathbf{P}}_{{L}}^{{{sch}}} }}{{\partial {{\textbf{x}}}}}} & {\frac{{\partial {\mathbf{P}}_{{{ag}}}^{ * } }}{{\partial {{\textbf{x}}}}}} & {\frac{{\partial {\mathbf{Q}}_{{g}}^{{{\rm sche}}} }}{{\partial {{\textbf{x}}}}}} & {\frac{{\partial {\mathbf{Q}}_{{L}}^{{{\rm sche}}} }}{{\partial {{\textbf{x}}}}}} & {\frac{{\partial {\mathbf{Q}}_{{r}} }}{{\partial {{\textbf{x}}}}}} & {\frac{{\partial {\mathbf{P}}_{{{ag}}}^{{{dif}}} }}{{\partial {{\textbf{x}}}}}} & {\frac{{\partial {\mathbf{P}}_{{T}} }}{{\partial {{\textbf{x}}}}}} \\ \end{array} } \right]^{{{T}}} $$

$$ {\mathbf{P}}_{ag}^{dif} ({{\textbf{x}}}) = {\mathbf{P}}_{ag}^{ \otimes } ({{\textbf{x}}}) - {\mathbf{P}}_{ag}^{ \bullet } ({{\textbf{x}}}) $$

${\textbf{J}_1}$ is conventional Jacobian matrix, ${\textbf{J}_2}$ is DG-load related Jacobian matrix. Some elements of ${\textbf{J}_2}$ related to IM can be found in [21]. The derivative term ${{\partial P_{\rm loss} } \mathord{\left/ {\vphantom {{\partial P_{\rm loss} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}}$ in ${\textbf{J}_2}$ can be simply illustrated. Let ${\mathbf{V}}_{{f}}$, ${\mathbf{V}}_{t}$ and ${\varvec{\uptheta }}_{f}$, ${\varvec{\uptheta }}_{t}$ are denoted column vector of voltage and angle, from and to ends, respectively. ${\mathbf{R}}$,${\mathbf{X}}$, ${\mathbf{B}}_{c}$, ${\mathbf{T}}_{ap}$ are denoted column vector of branch resistance, reactance, susceptance, tap ratio, respectively. The power loss in (19) can be written in column vector by,

$$ {\mathbf{P}}_{\rm loss} = {\mathbf{R}}\left( {({{\mathbf{V}}}_{f} /{{\mathbf{T}}}_{ap} )^{2} + ({{\mathbf{V}}}_{t} )^{2} - 2({{\mathbf{V}}}_{f} /{{\mathbf{T}}}_{ap} ){{\mathbf{V}}}_{t} \cos ({\varvec{\uptheta }}_{f} - {\varvec{\uptheta }}_{t} )} \right)/\Delta $$

(26)

where $\Delta = {{\mathbf{R}}}^{2} + ({{\mathbf{X}}}(1 + {\rm d}f))^{2}$. The derivative of power loss with respect to the frequency variation is given by,

$$ {{\partial {{\mathbf{P}}}_{\rm loss} } \mathord{\left/ {\vphantom {{\partial {{\mathbf{P}}}_{\rm loss} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}} = - 2{{\mathbf{P}}}_{\rm loss} {{\mathbf{X}}}^{2} (1 + {\rm d}f)/\Delta $$

(27)

The derivative of active power of DG and load with respect to the frequency deviation is given by,

$$ {{\partial {{\mathbf{P}}}_{g} } \mathord{\left/ {\vphantom {{\partial {{P}}_{g} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}} = - 1/{{\mathbf{m}}}_{p} $$

(28)

$$ {{\partial {{\mathbf{P}}}_{L} } \mathord{\left/ {\vphantom {{\partial {{\mathbf{P}}}_{L} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}} = - {{\mathbf{P}}}_{0} {{\mathbf{V}}}^{\mathbf{\upalpha }} {{\mathbf{K}}}_{pf} $$

(29)

The derivative of air-gap power with respect to the frequency deviation is given by,

$$ \frac{{\partial {{\mathbf{P}}}_{{{ag}}}^{ * } }}{\partial {\rm d}f} = - 2{{\mathbf{R}}}_{s} \left( {{{{\textbf{E}}^{\prime\prime}}}_{m} } \right)^{2} \left( {{{\mathbf{R}}}_{R}^{ - 3} {{\partial {{\mathbf{R}}}_{R} } \mathord{\left/ {\vphantom {{\partial {{R}}_{R} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}} + ({{\mathbf{X}}}_{M}^{ - 1} + {{\mathbf{X}}}_{R}^{ - 1} )\left( {{{\partial ({1 \mathord{\left/ {\vphantom {1 {{{\mathbf{X}}}_{M} }}} \right. \kern-\nulldelimiterspace} {{{\mathbf{X}}}_{M} }})} \mathord{\left/ {\vphantom {{\partial ({1 \mathord{\left/ {\vphantom {1 {{{\mathbf{X}}}_{M} }}} \right. \kern-\nulldelimiterspace} {{{\mathbf{X}}}_{M} }})} {\partial {\rm d}f + {{\mathbf{X}}}_{R}^{ - 2} {{\partial {{\mathbf{X}}}_{R} } \mathord{\left/ {\vphantom {{\partial {{\mathbf{X}}}_{R} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}}}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f + {{\mathbf{X}}}_{R}^{ - 2} {{\partial {{\mathbf{X}}}_{R} } \mathord{\left/ {\vphantom {{\partial {{\mathbf{X}}}_{R} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}}}}} \right)} \right) $$

(30)

where the column entries of ${{\partial {{\mathbf{R}}}_{R} } \mathord{\left/ {\vphantom {{\partial {{\mathbf{X}}}_{R} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}}$,${{\partial {{\mathbf{X}}}_{R} } \mathord{\left/ {\vphantom {{\partial {{\mathbf{X}}}_{R} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}}$ and ${{\partial ({1 \mathord{\left/ {\vphantom {1 {{{\mathbf{X}}}_{M} }}} \right. \kern-\nulldelimiterspace} {{{\mathbf{X}}}_{M} }})} \mathord{\left/ {\vphantom {{\partial ({1 \mathord{\left/ {\vphantom {1 {{{\mathbf{X}}}_{M} }}} \right. \kern-\nulldelimiterspace} {{{\mathbf{X}}}_{M} }})} {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}}$ are given by,

$$ {{\partial R_{R} } \mathord{\left/ {\vphantom {{\partial R_{R} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}} = k\left( {{{\partial r_{r}^{2} } \mathord{\left/ {\vphantom {{\partial r_{r}^{2} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}} + {{\partial x_{r}^{2} } \mathord{\left/ {\vphantom {{\partial x_{r}^{2} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}}} \right) - {{(R_{R} /r_{r} )\partial r_{r} } \mathord{\left/ {\vphantom {{(R_{R} /r_{r} )\partial r_{r} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}} $$

$$ {{\partial X_{R} } \mathord{\left/ {\vphantom {{\partial X_{R} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}} = k\left( {{{\partial r_{r}^{2} } \mathord{\left/ {\vphantom {{\partial r_{r}^{2} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}} + {{\partial x_{r}^{2} } \mathord{\left/ {\vphantom {{\partial x_{r}^{2} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}}} \right) - {{(X_{R} /x_{r} )\partial x_{r} } \mathord{\left/ {\vphantom {{(X_{R} /x_{r} )\partial x_{r} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}} $$

$$ {{\partial r_{r}^{2} } \mathord{\left/ {\vphantom {{\partial r_{r}^{2} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}} = 2r_{r} {{\partial r_{r} } \mathord{\left/ {\vphantom {{\partial r_{r} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}} $$

$$ {{\partial x_{r}^{2} } \mathord{\left/ {\vphantom {{\partial x_{r}^{2} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}} = 2x_{r} {{\partial x_{r} } \mathord{\left/ {\vphantom {{\partial x_{r} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}} $$

$$ {{\partial r_{r} } \mathord{\left/ {\vphantom {{\partial r_{r} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}} = \frac{{ - r_{r} \omega_{r} }}{{(1 + {\rm d}f)(1 + {\rm d}f - \omega_{r} )}} + \frac{{2B(1 + {\rm d}f) - 2r_{r} F(1 + {\rm d}f - \omega_{r} )}}{{E + F(1 + {\rm d}f - \omega_{r} )^{2} }} $$

$$ {{\partial x_{r} } \mathord{\left/ {\vphantom {{\partial x_{r} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}} = \frac{{C + D(1 + {\rm d}f - \omega_{r} )^{2} }}{{E + F(1 + {\rm d}f - \omega_{r} )^{2} }} - X + \frac{{2(1 + {\rm d}f - \omega_{r} )\left( {D(1 + {\rm d}f) - F(x_{r} + X(1 + {\rm d}f))} \right)}}{{E + F(1 + {\rm d}f - \omega_{r} )^{2} }} $$

$$ {{\partial (1/X_{m} )} \mathord{\left/ {\vphantom {{\partial (1/X_{m} )} {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}} = - X_{rr} /((1 + {\rm d}f)^{2} X_{m}^{2} ) $$

Finally, the derivative of power loss with respect to the frequency deviation is given by,

$$ {{\partial {\mathbf{\textit{{P}}}}_{\rm loss} } \mathord{\left/ {\vphantom {{\partial {\mathbf{P}}_{\rm loss} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}} = \sum {{{\partial {{\mathbf{P}}}_{g} } \mathord{\left/ {\vphantom {{\partial {{\mathbf{P}}}_{g} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}}} + \sum {{{\partial {{\mathbf{P}}}_{L} } \mathord{\left/ {\vphantom {{\partial {{\mathbf{P}}}_{L} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}}} + \sum {{{\partial {{\mathbf{P}}}_{ag} } \mathord{\left/ {\vphantom {{\partial {{\mathbf{P}}}_{ag} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}} - } \sum {{{\partial {{\mathbf{P}}}_{\rm loss} } \mathord{\left/ {\vphantom {{\partial {{\mathbf{P}}}_{\rm loss} } {\partial {\rm d}f}}} \right. \kern-\nulldelimiterspace} {\partial {\rm d}f}}} $$

1.2 Appendix B

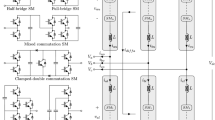
Appendix B provides a detail of the tested motors in physical unit as shown in Fig. 3 and 4.

	Rs	Lls	Rr1	Llr1	Rr2	Llr2	Lm	Nr	V
Single-cage induction motor
3HP	0.435	0.002	0.816	0.002	-	-	0.0693	1710	220
3HP	3.5	0.0063	3.16	0.0068	-	-	0.2667	1420	400
3 kW	2.89	0.1342	2.39	0.011	-	-	0.0241	1390	400
Type-1 double-cage induction motor
8 kW	0.382	0.00413	0.7644	0.00843	3.54	0.00413	0.0767	960	400
Type-2 double-cage induction motor
8 kW	0.382	0.00413	2.5	0.00343	0.7644	0.0043	0.0767	963	400